Standard Deviation

Standard Deviation

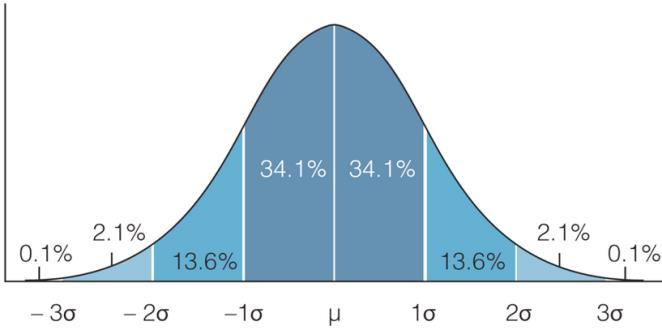
Standard deviation (σ) provides a tool to determine if measurements are statistically equivalent and differ by only random error present in the data.

If only random error is present, the measurements should adopt a Gaussian distribution around the true value (μ). The probability that your measurement will be 1σ from the true value is 68.2 %, 2σ from the turn value 95.4 % and 3σ will be 99.6 %.

The difference in the measurements are not sufficiently different to state that they are uniquely different values.

To calculate a standard deviation for a set of equivalent measurements do the following:

1. Calculate the average value of the equivalent measurements.
2. Calculate the deviation of each measurement from the average (actual measurement – average measurement).
3. Calculate the variance of each measurement (square the deviation for each measurement).
4. Calculate the average variance of each measurement.
5. The standard deviation of the measurements is equal to the square root of the average variance.

The precision (significant figures) of the standard deviation is equal to that of the average measurement. For example, if the average measurement for a set of distance measurements = 15.01 m, the standard deviation is kept to the hundredths.

If the average of a series of measurements was 15.01 kg with a standard deviation of 0.03 kg. The overall result could be written as 15.01(3) kg. The standard deviation is generally recorded as the last significant digit of the measurement. What this means is that if two measurements were within ±3σ of each other (in this case ±0.09 kg) the two measurements would have to be considered statistically equivalent. In other words, the precision of the measurement was not sufficient to tell them apart!

Comparing measured values using significant figures.

Three objects possess the following lengths 15.01(3) kg, 15.11(4) kg and 14.91(1) kg. Are these statistically different masses?

To compare values with different standard deviations, you must first calculate the overall standard deviation of the measurement:

The overall standard deviation is the square root of the sum of the squares of the standard deviation of the measurements.

1. To compare 15.01(3) kg with 15.11(4) kg, the combined standard deviation (σ12) can be calculated as:
   
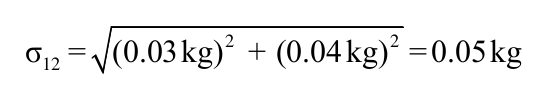
   Therefore. the measurements are identical if they are within ±3σ. To determine this ∆/σ should be calculated (the number of standard deviations difference between the two measurements.
   
   
   Seeing the measurements are only 2σ different the measurements must be considered statistically identical.
   
   
   Take Note

   The measuring device cannot differentiate between the two measurements. The difference in the measurements is explained by random variation in the measurement.

    

   
   
   
2. ii) Compare 15.11(4) kg with 14.91(1) kg.
   
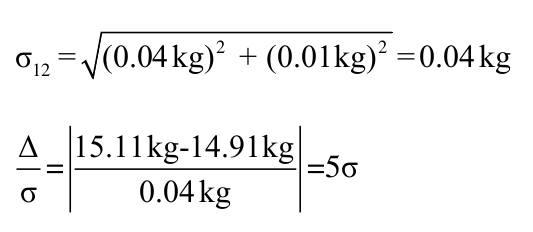
   These measurements are 5σ different are therefore statistically different.
   
   
   Take Note

   Notice, the higher precision measurements (smaller standard deviation) are better able to distinguish between different values.
   The moral to the story is, learn how to collect data accurately and precisely!